
G = members of the red circle but of neither the green nor the blue circlesĭepending on context, there may also be a eighth discrete region, those elements that are not members of any of the three set. This diagram has at least seven discrete regionsī = members of the green and blue circles, but not the red circleĬ = members of the green and red circles, but not the blue circleĭ = members of the blue and red circles, but not the green circleĮ = members of the green circle but of neither the blue nor the red circlesį = members of the blue circle but of neither the green nor the red circles If there are three overlapping categories, we use a three-circle Venn diagram: Once you know the value of each individual discrete region, you will be able to answer any question about the number in any particular grouping. In general, you work from the center outward, figuring out one discrete region after another. If you are told there are 70 members in the right circle, and 20 members in the overlap, then you would know B + C = 70 and B = 20, so from that you could deduce C = 50, the number of elements that are just in the portion of the circle labeled C. Typically, the problem will only give us information about totals - the total number of elements altogether, the total number in each circle, and the overlap. There may also be a fourth discrete region, those elements that are not members of any set. This diagram contains three discrete regions:Ī = those elements in just the left circleī = those element in both categories, in the overlapĬ = those elements in just the right circle If you have two overlapping sets, you need a two-circle Venn diagram: Venn diagrams are the best method for untangling overlapping sets. This is exactly the situation of the practice questions posed above. As it happens, I am a member of exactly two of those categories. Those are three overlapping sets - any particular adult resident of the SF Bay Area many be a member of none, one, two, or all three of those three categories. For example, in the SF Bay Area, many adult residents were born out of state - many, but not all many adult residents have a college education - many, but not all and many adult residents are SF Giants fans - many, but not all.

Things get more interesting if there are two or more overlapping sets. Ordinarily, there’s nothing particular challenging if there’s only one set: some of the people are in that set, whatever it is, and the rest aren’t. Non-numerical sets, sets in which the members are people or cars or companies, are the stuff of tricky word problems. Numerical sets can be handled with statistical calculations. You can read more about those calculations at this post. When all the members of the set are numbers, typical questions involve computations like the mean or the median: here, the mean (or average) of set A is 4, and the median of A is 3. That notation denotes set A with five numerical members. When the members of a set are all numbers, then we use “set notation”, which consists of brackets. A set is simply a collection of objects or elements. The idea of a set is, in some sense, the most fundamental idea in all of mathematics.
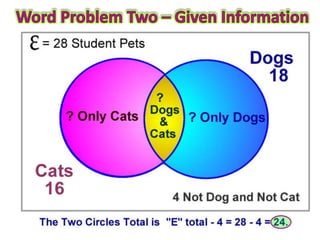
How many students participate in only the drama? Twenty-five students are just in the chorus, not in anything else.
Furthermore, 37 students are in both the Band and Chorus, 20 are in both the Band and the Drama, and 8 students are in all three groups. There are 70 students in Band, 73 in the Chorus, and 45 in the Drama. Students must participate in at least one, and may participate in two or even in all three. How many houses in the development have neither a two-car garage nor an in-the-ground swimming pool?Ģ) A certain school has three performing arts extracurricular activities: Band, Chorus, or Drama. Learn this technique to master set questions of GMAT Math word problems.įirst, try these challenging practice questions.ġ) Of the 80 houses in a development, 50 have a two-car garage, 40 have an in-the-ground swimming pool, and 35 have both a two-car garage and an in-the-ground swimming pool. By Mike MᶜGarry on Aug, UPDATED ON April 20, 2019, in GMAT Word Problems


 0 kommentar(er)
0 kommentar(er)
